Question

Type the correct answer in each box.

Find the elements of matrix A.

help PLS!!

Answer 1

a = 2

b = 11

c = 11

d = -2

Explanation:

`\sf \left[\begin{array}{cc}a&b\\c&d \end{array}\right] *\left[\begin{array}{ccc} 22&11&7\\ 6&-9&-15 \end{array}\right] =\left[\begin{array}{ccc} 22a+6b&11a-9b&7a-15b\\22c+6d&11c-9d&7c-15d \end{array}\right]`

`\sf \left[\begin{array}{ccc} 22a+6b&11a-9b&7a-15b\\22c+6d&11c-9d&7c-15d \\ \end{array}\right] =\left[\begin{array}{ccc} 110&-77&-151\\230&139&107 \\ \end{array}\right]`

On comparing to right side, we get,

22a + 6b = 110

Divide the entire equation by 2

11a + 3b = 55 ------------(I)

11a - 9b = -77 -------------(II)

Subtract equation (II) from (I)

11a + 3b = 55

11a - 9b = -77

- + + {Now subtract}

12b = 132

b = 132/12

`\sf \boxed{\bf \ b = 11}`

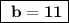
Substitute the value of b in eqaution (I)

11a + 3*11 = 55

11a + 33 = 55

11a = 55 - 33

11a = 22

a = 22/11

`\sf \boxed{\bf \ a = 2}`

22c + 6d = 230

Divide by 2

11c + 3d = 115 -----------------(III)

11c - 9d = -139 ------------------(IV)

Subtract (IV) from (III)

11c + 3d = 115

11c - 9d = 139

- + -

12d = -24

d = -24/12

`\sf \boxed{\bf \ d = -2}`

Plugin d = - 2 in equation (III)

11c + 3*(-2) = 115

11c - 6 = 115

11c = 115 + 6

11c = 121

c = 121/11

`\sf \boxed{\bf \ c = 11}`