Answer:
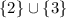
Step-by-step explanation:
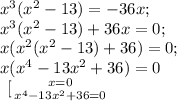
We have two equalities.
Let us analyze the latter.
Let
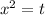 on condition that
on condition that
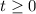 , then:
, then:
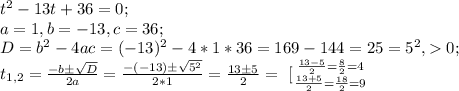
Substitute
 back for
back for
 , keep in mind that
, keep in mind that
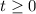 .
.
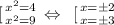
Therefore,
 is our set of solutions. Given the condition that we need our variable to be more than zero, our answer is
is our set of solutions. Given the condition that we need our variable to be more than zero, our answer is
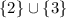 .
.