Answer:

Explanation:
First we will find the area of Square PQRS.
Area of Square PQRS = Length x Width = 21 x 21
=

Next we will found the Area of Semicircles PS and QR.
Note: Area of Semicircle PS = Area of Semicircle QR
Area of Semicircle =

Total Area of Semicircles PS and QR combined =
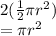
We know that the diameter of PS = QR = 21 cm (due to the length of the square)
Radius = Half of Diameter = 0.5 x 21cm = 10.5cm
Total Area of Semicircles PS and QR =
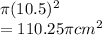
Finally,
Area of Shaded Region = Area of Rectangle PQRS - Total Area of Semicircles PS and QR
=

In this case , you can choose the nearest answer as there might be some rounding differences.