Answer:
1. 180 degrees
2. 4
3. 8π
4. 8:1
5. 12.5 feet
6. 3 revolutions
7. π/2
8. 64π
Explanation:
1. For the first question, we are given that there are 120 degrees in 1/3 of a circle. This makes sense because a complete circle makes up 360 degrees (3*(1/3)). Two figure out how many more degrees there are in 5/6 circle, we first need to figure out how many degrees are in 1/6 of a circle and then 5/6 of a circle. If there are 120 degrees in 1/3 of a circle, there are 120/2 or 60 degrees in 1/6 of a circle (1/6 is half of 1/3). If we multiply this by 5 (because 1/5 * 5 = 5/6), we get that 5/6 of a circle is 60*5 or 300 degrees. That being said, the question is asking us how many more degrees there are in 5/6 circle than 1/3. We know that there are 120 degrees in 1/3 of a circle, so we can do 300 - 120 to figure out the answer, which comes out to be 180 degrees.
2. For the second question, we are given that the area of the circle is 4π. We know that the formula for the area of a circle is
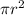, which means the radius squared of the circle given is 4 (divide by π). If we take the square root of 4, we get the radius of our circle to be 2. Now, all we have to do is figure out the diameter. The radius is the distance between a point on the circle and the center. The diameter is just double this distance because it is the distance between one point on a circle and another point on the exact opposite side (the diameter line segment goes through the center). Since the radius is 2, the diameter has to be 4.
3. For the third question, we are given that the radius of a circle is 4 and we need to figure out the circumference (q). We know that the formula for the circumference of a circle is πd, or 2πr (r is the radius). We can plug the known radius into the equation and solve for the circumference. If we plug in r = 4, we get that the circumference (q) is 2(4)(π), or 8π.
4. For the fourth question, we are given that the radius of circle Q is 4 times the size of the diameter of circle R. We know that the diameter of any circle is just double the size of the radius. This means that, if the radius of circle Q is four times the diameter of circle R, the diameter of circle Q is 4(2) or 8 times the size of the diameter of circle R. This can be represented in ratio form as 8:1 (as in, if the diameter of circle R was 1, the diameter of circle Q would be 8, which is 8 times the size).
5. For the fifth question, the distance traveled in one revolution of a circle is the same as the circumference of the circle. You can imagine a circle and a point on it that is touching the ground. The circumference of this circle (the length of the circle) is the same as the distance traveled between the time the point touches the ground to the time it touches the ground again. Please feel free to let me know if this doesn't make sense. I will try to draw it out. Anyway, we are given the diameter of this wheel (4 feet), and we know that the circumference of a circle is π*d, so the circumference, in this case, would be 4π, which is approximately 12.5 feet.
6. For the sixth question, we just need to figure out the circumferences of the two wheels and compare them. If we go back to the fifth question, we know that the distance traveled by a wheel in one rotation is the same as its circumference. Let's say that the radius of the rear wheel is x. This means that the radius of the front wheel is 3x. The circumference of a circle is 2πr, which means the circumference of the front wheel is 6πx and the circumference of the rear wheel is 2πx. The circumference of the front wheel is 3 times the size of the rear wheel's circumference, which means that it will take 3 revolutions of the rear wheel to complete 1 revolution of the front wheel
7. For the seventh question, if AD is a half circle, that means the side of the square that connects A and D is the diameter of the circle. We know that this diameter is 2 because the length of each side of the square is 2. If the diameter is 2, that means the radius of the half-circle is half of that, or 1. The area of a circle is πr^2, so this circle has a radius of π (r^2 is 1). A half-circle has half of the area of a full circle, so the area of this half-circle would be π/2.
8. For question 8, we know that the circumference of circle A is 8π, and the circumference of any circle is 2πr. This means the radius of circle A is half of 8, or 4. We are given that the radius of circle B is twice the radius of circle A, which means the radius of circle B is 8. The area of a circle is πr^2, and r^2 for circle B is 8^2 or 64. Therefore, the area of circle B is 64π.