Question

A sample of 50 potential customers was asked to use your new product and the product of the leading competitor. After one week, they were asked to indicate which product they preferred. In the sample, 30 potential customers said that they preferred your product. (a) Find the sample proportion. (5 points) (b) Find the 95% confidence interval for the proportion of potential customers who prefer your product. (10 points) (c) If you want the 95% maximum likely error to be 0.08 or less, what would you choose for a sample size

Answer 1

a) The sample proportion is of 0.6.

b) The 95% confidence interval for the proportion of potential customers who prefer your product is (0.4642, 0.7358).

c) A sample size of 145 is needed.

Explanation:

(a) Find the sample proportion.

30 out of 50. So

`\pi = (30)/(50) = 0.6`

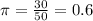
The sample proportion is of 0.6.

(b) Find the 95% confidence interval for the proportion of potential customers who prefer your product.

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

For this problem, we have that:

`n = 50, \pi = 0.6`

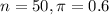
95% confidence level

So
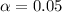`\alpha = 0.05`, z is the value of Z that has a pvalue of
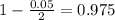`1 - (0.05)/(2) = 0.975`, so
`Z = 1.96`.

The lower limit of this interval is:

`\pi - z\sqrt{(\pi(1-\pi))/(n)} = 0.6 - 1.96\sqrt{(0.6*0.4)/(50)} = 0.4642`

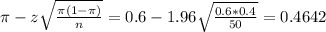
The upper limit of this interval is:

`\pi + z\sqrt{(\pi(1-\pi))/(n)} = 0.6 + 1.96\sqrt{(0.6*0.4)/(50)} = 0.7358`

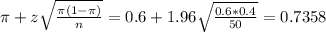
The 95% confidence interval for the proportion of potential customers who prefer your product is (0.4642, 0.7358).

(c) If you want the 95% maximum likely error to be 0.08 or less, what would you choose for a sample size

The margin of error is:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

We need a sample size of n, and n is found when M = 0.08. So

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.08 = 1.96\sqrt{(0.6*0.4)/(n)}`

`0.08√(n) = 1.96*sqrt{0.6*0.4}`

`√(n) = \frac{1.96*sqrt{0.6*0.4}}{0.08}`

`(√(n))^2 = (\frac{1.96*sqrt{0.6*0.4}}{0.08})^2`

`n = 144.1`

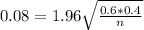
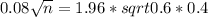
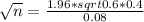
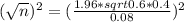
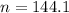
Rounding up

A sample size of 145 is needed.