Given:
The vertices of a triangle are R(3, 7), S(-5, -2), and T(3, -5).
To find:
The vertices of the triangle after a reflection over x = -3 and plot the triangle and its image on the graph.
Solution:
If a figure reflected across the line x=a, then
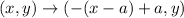
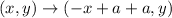
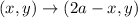
The triangle after a reflection over x = -3. So, the rule of reflection is
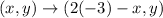
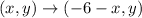
The vertices of triangle after reflection are

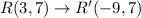
Similarly,
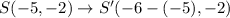
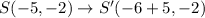
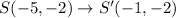
And,
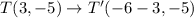
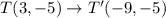
Therefore, the vertices of triangle after reflection over x=-3 are R'(-9,7), S'(-1,-2) and T'(-3,-5).