Question

We have a parallel-plate capacitor with plates of metal each having a width W and a length L. The plates are separated by the distance d. Assume that L and W are both much larger than d. The maximum voltage that can be applied is limited to V max =K d, in which K is called the breakdown strength of the dielectric. Derive an expression for the maximum energy that can be stored in the capacitor in terms of K and the volume of the dielectric. If we want to store the maximum energy per unit volume, does it matter what values are chosen for L, W, and d? What parameters are important?

Answer 1

max energy =
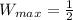`W_(max) = (1)/(2)`*εo*εr*k^2

Step-by-step explanation:

Given data:

weight of plates = W

length of plates = L

distance of separation = d

max voltage ( Vmax ) = Kd

Area ( A ) = WL

The values chosen for L, W, and d matters, although the maximum energy stored in the capacitor is independent of L, W, and d. but at a constant volume and a larger value for W and L which is > d, the value of the dielectric (εrK^2 ) should be a larger value '

The important parameters are : εrK^2 , k , d and Area

attached below is the remaining part of the solution