Question

To determine whether the pipe welds in a nuclear power plant meet specifications, a random sample of welds is selected, and tests are conducted on each weld in the sample. Weld strength is measured as the force required to break the weld. Suppose the specifications state that mean strength of welds should exceed 100 lb/in2; the inspection team decides to test

H0:μ=100 versus Ha:μ>100.
Explain why it might be preferable to use this Ha rather than μ<100.
A. We want to determine if there is significant evidence that the mean strength of welds differs from 100 lb/in2. The current hypotheses correctly place the burden of proof on those who wish to assert that the specification is not satisfied.
B. We want to determine if there is significant evidence that the mean strength of welds is less than 100 lb/in2. The current hypotheses correctly place the burden of proof on those who wish to assert that the specification is not satisfied.
C. We want to determine if there is significant evidence that the mean strength of welds exceeds 100 lb/in2. The current hypotheses correctly place the burden of proof on those who wish to assert that the specification is satisfied.
D. We want to determine if there is significant evidence that the mean strength of welds equals 100 lb/in2. The current hypotheses correctly place the burden of proof on those who wish to assert that the specification is satisfied.

Answer 1

C. We want to determine if there is significant evidence that the mean strength of welds exceeds 100 lb/in2. The current hypotheses correctly place the burden of proof on those who wish to assert that the specification is satisfied.

Explanation:

Given

`\mu = 100`

`H_0:\mu = 100\\\\H_a:\mu > 100`

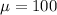
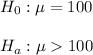
Required

State why it is preferred to
`Ha: \mu < 100`

From the question, we understand that the initial test is the force required to break the weld.

This represents the null hypothesis

Because the required force can not be less than the strength of the weld (it must match the force keeping the weld), the null hypothesis is represented as:

`H_0:\mu = 100`

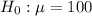
Reading the question further;

The specification requires that the mean strength should exceed 100

In inequalities, exceeds means greater than, and it is represented with >

So, the null hypothesis is rightly represented as:

`H_a:\mu > 100`

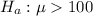
Hence: (c) is true