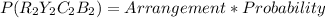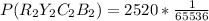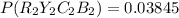Question

On each turn of the knob, a gumball machine is equally likely to dispense a red, yellow, green or blue gumball, independent from turn to turn. After eight turns, what is the probability P[R2Y2C2B2] that you have received 2 red, 2 yellow, 2 green and 2 blue gumballs?

Answer 1

`P(R_2Y_2C_2B_2) = 0.03845`

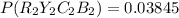
Explanation:

Given

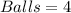`Balls = 4` --- [R, Y, C and B]

`Turns = 8`

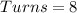
Required

`P(R_2Y_2C_2B_2)`

Since each of the 4 balls are equally likely, their probability is:

`P(R) = P(Y) = P(C) = P(B) = (1)/(4)`

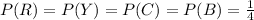
From
`P(R_2Y_2C_2B_2)`, we have:

`R = Y = C = B = 2`

`Total = 8`

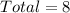
So, the total arrangement of the 8 balls is:

`Arrangement = (8!)/(2!2!2!2!)`

`Arrangement = (40320)/(2*2*2*2)`

`Arrangement = (40320)/(16)`

`Arrangement = 2520`

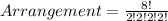
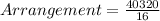
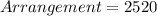
The individual probability of each ball, when put together is

`Probability = P(R)^2 * P(Y)^2 * P(C)^2 * P(B)^2`

`Probability = (1/4)^2 *(1/4)^2 *(1/4)^2 *(1/4)^2`

`Probability = (1/16) *(1/16) *(1/16) *(1/16)`

`Probability = (1)/(65536)`

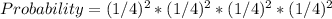
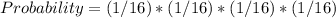
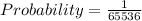
Lastly:

`P(R_2Y_2C_2B_2) = Arrangement * Probability`

`P(R_2Y_2C_2B_2) = 2520 * (1)/(65536)`

`P(R_2Y_2C_2B_2) = (2520 )/(65536)`

`P(R_2Y_2C_2B_2) = 0.03845`