Answer:
The value of the test statistic is 2.47.
Explanation:
The test statistic is:
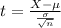
In which X is the sample mean,
 is the expected mean,
is the expected mean,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
For a proportion p, we have that:

A political study took a sample of 900 voters in the town and found that 42% of the residents favored annexation.
This means that
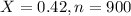
Using the data, a political strategist wants to test the claim that the percentage of residents who favor annexation is above 38%
This means that the expected is
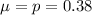
So
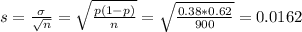
Find the value of the test statistic
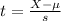
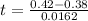
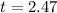
The value of the test statistic is 2.47.