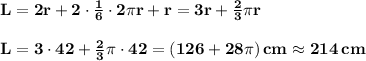Answer:
(a) 44 cm
(b) 214 cm
Explanation:
(a)
The figure perimeter it is half of a circle with a diameter d₁=14 cm and two halfs of circle with a diameter d₂=14 cm÷2=7 cm.
The length of a circle is L₀ = πd, where d is the diameter.
Therefore:
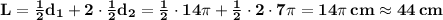
(b)
A full circle it is 360°, so the circle sector of 60° is
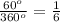 of the circle. So the arc of 60° is ¹/₆ of the full circle length.
of the circle. So the arc of 60° is ¹/₆ of the full circle length.
The figure is an equilateral triangle and two 60°-sectors of circles with radiuses of length of the triangle's side (r=42 cm).
Its perimetr it's two radiuses, two arcs of 60° and one side of the triangle.
The length of a circle is L₀ = 2πr, where r is a radius.
Therefore: