Answer:
λ = 5.656 x 10⁻⁷ m = 565.6 nm
Step-by-step explanation:
Using the formula of fringe spacing from the Young's Double Slit experiment, which is given as follows:
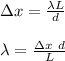
where,
λ = wavelength = ?
Δx = fringe spacing = 1.6 cm = 0.016 m
L = Distance between slits and screen = 4.95 m
d = slit separation = 0.175 mm = 0.000175 m
Therefore,

λ = 5.656 x 10⁻⁷ m = 565.6 nm