Answer:
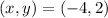
Explanation:
Given
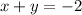
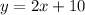
Required
Determine the point of intersection of both equations (if graphed)
To do this, we solve for x and y
Substitute 2x + 10 for y in the first equation
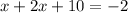


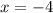
Substitute
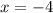 in the second equation
in the second equation
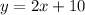
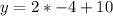
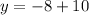
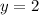
So, the point of intersection is:
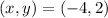
See attachment for graph