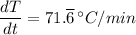Question

When an amount of heat Q (in kcal) is added to a unit mass (kg) of a

substance, the temperature rises by an amount T (°C). The quantity dQ/dT, called
specific heat, is 0.18 (kcal/°C) for glass. If dQ/dt = 12.9 kcal/min for a 1 kg sample
of glass at 20.0 °C, find dT/dt for this same sample. (Note: T is for temperature, t is
for time.)

Answer 1

The change in temperature per minute for the sample, dT/dt is 71.
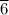`\overline {6}` °C/min

Explanation:

The given parameters of the question are;

The specific heat capacity for glass, dQ/dT = 0.18 (kcal/°C)

The heat transfer rate for 1 kg of glass at 20.0 °C, dQ/dt = 12.9 kcal/min

Given that both dQ/dT and dQ/dt are known, we have;

`(dQ)/(dT) = 0.18 \, (kcal/ ^(\circ) C)`

`(dQ)/(dt) = 12.9 \, (kcal/ min)`

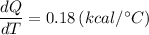
Therefore, we get;

`((dQ)/(dt) )/((dQ)/(dT) ) = {(dQ)/(dt) } * (dT)/(dQ) = (dT)/(dt)`

`(dT)/(dt) = ((dQ)/(dt) )/((dQ)/(dT) ) = (12.9 \, kcal / min )/(0.18 \, kcal/ ^(\circ) C ) = 71.\overline 6 \, ^(\circ ) C/min`

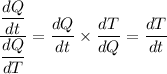
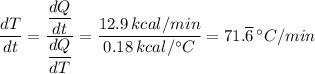
For the sample, we have the change in temperature per minute, dT/dt, presented as follows;

`(dT)/(dt) = 71.\overline 6 \, ^(\circ ) C/min`