Answer: Choice C.
The difference between our sample mean and our hypothesized mean was statistically significant.
=========================================================
Step-by-step explanation:
The null hypothesis is always set up in a way to state the following: "The mean mu is equal to some value"
The mu is a greek letter often used as the population mean symbol. The actual symbol itself looks like this
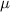
When we reject the null, we reject the idea that the mu is whatever stated value we said in the null. So we must go with the alternative hypothesis.
The sample mean we computed (xbar) is different enough from the mu value that we're able to make such a rejection. We consider this statistically significant, which is another way of saying that such a difference is not simply due to random chance.