Question

2. Cory makes a map of his favorite park, using a coordinate system with units of yards. The old oak tree is at position (1, 10) and the granite boulder is at position (-5, 9). How far apart are the old oak tree and the granite boulder? Round to the nearest tenth if necessary

Answer 1

Answer: The old oak tree and the granite boulder are
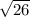`√(26)` units apart.

Explanation:

The distance between points (a,b) and (c,d) is given by :-

`d=√((c-a)^2+(d-b)^2)`

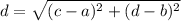
Given: The position of oak tree = (1, 10)

The position of granite boulder = (-5,9)

The distance between oak tree and granite boulder =
`√((10-9)^2+(1-(-5))^2)`

`=√((1)^2+(1+5)^2)\\\\=√(1+25)\\\\=√(26)\text{ units}`

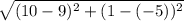
Hence, the old oak tree and the granite boulder are
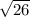`√(26)` units apart.