Final answer:
The equilibrium position for a -3.0nC charge between charges of 7.2nC and 6.7nC can be found by applying Coulomb's Law and equating the magnitudes of the electrostatic forces from both charges to be equal.
Step-by-step explanation:
To find the equilibrium position for a -3.0nC charge placed between charges of 7.2nC and 6.7nC, which are 32 cm apart, one must apply Coulomb's Law. The equilibrium point is where the net electrostatic force on the -3.0nC charge is zero. This requires setting the magnitude of the force from each charge on the test charge to be equal, considering the distance x from the 7.2nC charge as the variable.
The formula to calculate the forces is
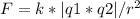, where k is Coulomb's constant, q1 and q2 are the charges, and r is the distance between the charges.
The problem becomes a matter of finding x such that F1 = F2, where F1 is the force due to the 7.2nC charge and F2 is the force due to the 6.7nC charge. One must consider both the magnitudes and the directions of the forces due to the nature of attraction and repulsion between charges.