Question

In the diagram below of circle 0, GO = 8 and

mZGOJ= 60°.
G
8
60°
What is the area, in terms of A, of the shaded
region?

Answer 1

Since the radius of this circle is 8 inches, the area of a sector, in terms of π, bounded by a 300° arc is: D. 160π/3 square units.

In Mathematics and Geometry, the area of a sector can be calculated by using the following formula:

Area of sector = π
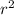`r^2` × θ/360

Where:

• r represents the radius of a circle.
• θ represents the central angle.

Note: The measure of an intercepted arc is equal to the central angle of a circle.

θ = 360 - 60

θ = 300°

By substituting the given parameters into the area of a sector formula, we have the following;

Area of sector = π
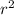`r^2` × θ/360

Area of sector = π ×
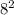`8^2` × 300/360

Area of sector = π × 64 × 5/6

Area of sector = π × 32 × 5/3

Area of sector = 160π/3 square units.

Complete Question:

In the diagram below of circle 0O, GO=8 and m∠ GOJ=60^o. What is the area, in terms of x, of the shaded region? 1) 4π /3 2) 20π /3 3) 32π /3 4) 160π /3

Answer 2

`\frac{80\pi}`

Explanation:

Area of shaded region = θ/360 × πr²

Where,

θ = 360 - 60 = 300°

r = 8

Plug in the values

`area = (300)/(360) * \pi * 8²`

`= (5)/(6) * \pi * 64`

`= (5*\pi*64)/(6)`

`= \frac{5*\pi*16}`

`= \frac{80\pi}`