Question

Vector W is in standard position, and makes an angle of 210 degrees with the positive x-axis, it's magnitude is 8. Write W in component form (a,b), and in linear combination form, ai+bj.

Answer 1

`\vec W = -4√(3)\,\hat{i}-4\,\hat{j}`,
`\vec W = (-4√(3),-4)`

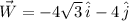Explanation:

If
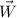`\vec W` is in standard position, then its direction is counterclockwise with respect to
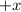`+x` semiaxis. A vector is defined by its magnitude and direction, that is:

`\vec W = \|\vec W\| \cdot (\cos \theta\,\hat{i}+\sin \theta \,\hat{j})` (1)

Where:

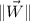`\|\vec W\|` - Magnitude, no unit.

`\theta` - Direction, measured in sexagesimal degrees.

If we know that
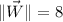`\|\vec W\| = 8` and
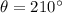`\theta =210^(\circ)`, then the resulting vector is:

`\vec W = 8\cdot (\cos 210^(\circ)\,\hat{i}+\sin 210^(\circ)\,\hat{j})`

`\vec W = -4√(3)\,\hat{i}-4\,\hat{j}`

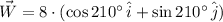
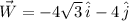
Which is also equivalent to:

`\vec W = (-4√(3),-4)`