Answer:
See below
Explanation:
Here we need to prove that ,

Imagine a right angled triangle with one of its acute angle as
 .
.
- The side opposite to this angle will be perpendicular .
- Also we know that ,
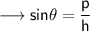
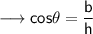
And by Pythagoras theorem ,
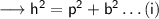
Where the symbols have their usual meaning.
Now , taking LHS ,

- Substituting the respective values,
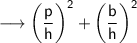
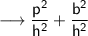
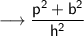
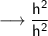
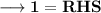
Since LHS = RHS ,
Hence Proved !
I hope this helps.