Answer:
Explanation:
1) x² + 6x + 11 = 0
a = coefficient of x² = 1
b = coefficient of x = 6
c = Constant = 11
Roots = (-b ± √b² - 4ac)/2a
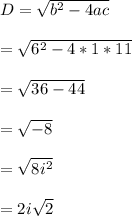
Roots =
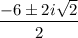

2) 2x² - 16x = 50
Divide the whole equation by 2
x² - 8x = 25
Divide the coefficient of x (i. e 8 ) by 2 = 8/2 = 4, Now add 4 to both sides of the equation
x² - 8x + 4 = 25 + 4
x² - 2*4*x + 2² = 29
(x - 2)² = 29
Take square root both side
