Question

A baker is building a rectangular solid box from cardboard to be able to safely deliver a birthday cake. The baker wants the volume of the delivery box to be 540 cubic inches. If the width of the delivery box is 3 inches longer than the length and the height is 4 inches longer than the length, what must the length of the delivery box be?

10 inches
9 inches
6 inches
3 inches

Answer 1

6 inches

Explanation:

I just took the test

Answer 2

C

Explanation:

The volume of a box (rectangular prism) is given by:

`\displaystyle V = \ell wh`

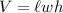
We are given that the desired volume is 540 cubic inches. The width is three inches longer than the length and the height is four inches longer than the length. Substitute:

`\displaystyle (540) = \ell(\ell + 3)(\ell + 4)`

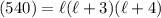
Solve for the length. Expand:

`\displaystyle \begin{aligned} 540 &= \ell (\ell^2 + 7\ell +12) \\ 540&= \ell ^3 + 7\ell^2 +12\ell \\ \ell^3 + 7\ell ^2 +12\ell -540 &= 0\end{aligned}`

We cannot solve by grouping, so we can consider using the Rational Root Theorem. Our possible roots are:

±1, ±2, ±3, ±4, ±5, ±6, ±9, ±10, ±12, ±15, ±18, ±20, ±27, ±30, ±36, ±45, ±54, ±60, ±90, ±108, ±135, ±180, ±270, and/or ±540.

(If you are allowed a graphing calculator, this is not necessary.)

Testing values, we see that:

`\displaystyle (6)^3 +7(6)^2 + 12(6) -540 \stackrel{\checkmark}{=} 0`

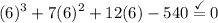
Hence, one factor is (x - 6).

By synthetic division (shown below), we can see that:

`\displaystyle \ell^3 + 7\ell^2 +12\ell -540 =(\ell -6)(\ell ^2 + 13\ell +90)`

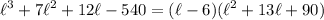
The second factor has no real solutions. Hence, our only solution is that l = 6.

In conclusion, our answer is C.