Question

Find the area of the trapezoid

Answer 1

Explanation:

6+11÷2×8=92 area of trapezoid

Answer 2

`\boxed{\sf{\green{68}}}` square centimeters.

Step-by-step explanation:

Here's the required formula to find the area of trapezoid :

`{\longrightarrow{\pmb{\sf{A = (b_1 + b_2)/(2) \cdot \: h}}}}`

• 
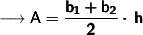
  `\purple\star` A = Area
• 
  `\purple\star` b₁ = 6 cm
• 
  `\purple\star` b₂ = 11 cm
• 
  `\purple\star` h = 8 cm

Substituting all the given values in the formula to find the area of trapezoid :

`{\longrightarrow{\sf{Area_((Trapezoid)) = (b_1 + b_2)/(2) \cdot \: h}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = (6 + 11)/(2) \cdot \: 8}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = (17)/(2) \cdot \: 8}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = (17)/(2) * 8}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = \frac{17}{\cancel{2}} * \cancel{8}}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = 17 * 4}}}`

`{\longrightarrow{\sf{Area_((Trapezoid)) = 68}}}`

`\star{\boxed{\sf{\pink{Area_((Trapezoid)) = 68 \: {cm}^(2)}}}}`

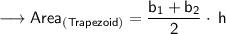
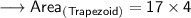
Hence, the area of trapezoid is 68 cm².

`\rule{300}{2.5}`