Answer:
The average hourly number of inches that the water level changes per day is about 0.0231 inches per hour.
Explanation:
The water level of a certain body of water is changing at a rate represented by the function:

Where W is measured in inches per hour and t represents hours since 12 A.M.
And we want to determine the average hourly number of inches that the water level changes for the lake in one day.
Recall that the average value of a function is given by:

Hence, the find the average hourly number of inches that the water level changes in one day, we simply need to find the average value of W from t = 0 to t = 24.
Substitute:
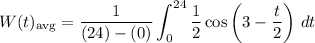
Simplify:

Evaluate using u-substitution. We can let:
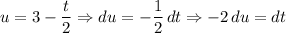
Hence:
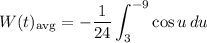
Evaluate:
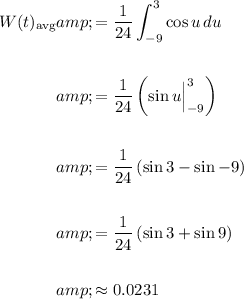
In conclusion, the average hourly number of inches that the water level changes per day is about 0.0231 inches per hour.