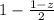Question

An electronic device factory is studying the length of life of the electronic components they produced. The manager selects two assembly lines and takes all samples on those two lines. He got a sample of 500 electronic components and records the length of life in the life test. A histogram indicates that the lifespans have moderate right skew. From the sample he found the average length of life was 200,000 hours and that the standard deviation was 1,000 hours. He wants to find the confidence interval for the average length of life of the electronic components they produced. Based on the information, what advice will you give to him

Answer 1

The confidence interval will be given by:

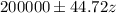`200000 \pm 44.72z`, in which z is related to the confidence level.

For a confidence level of x%, z is the value in the z-table that has a pvalue of
`1 - (1 - z)/(2)`

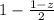
Explanation:

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

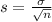For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

In the context of this problems:

It means that the sampling distributions of the sample mean of 500 components will be approximated normal, with mean 200,000 and standard deviation
`s = (1000)/(√(500)) = 44.72`

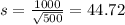
To build the confidence interval:

The confidence interval for the average length of life of the electronic components they produced will be given by:

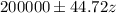`200000 \pm 44.72z`, in which z is related to the confidence level.

For a confidence level of x%, z is the value in the z-table that has a pvalue of
`1 - (1 - z)/(2)`