Answer:
You must deposit "$74,806.25" today.
Step-by-step explanation:
The given values are:
Periodic payment,
P = $10,300
Rate of interest,
r =
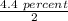
=
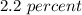
Number of periods,
n =

=
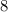
Now,
The PV of annuity will be:
=
![(P* [1 - (1 + r)^(-n)])/(r)](https://img.qammunity.org/2022/formulas/business/high-school/uu50jc1alhvks3mfh2pdfxy1lsetesbw3z.png)
On substituting the given values, we get
=
![(10,300* [1 - (1 + 2.2 \ percent)^(-8)])/(2.2 \ percent)](https://img.qammunity.org/2022/formulas/business/high-school/idygt46bycpyqn4jpsxv7b1yy60r7l79id.png)
=

=
 ($)
($)