Answer:
The answer is "
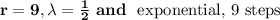 "
"
Explanation:
In point a:
We are aware of the random gamma variable X:
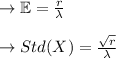
It is given:
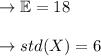
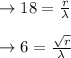
Substituting the value:

In point b:
When building the Erlang/Gammas distribution, these could reasonably be assumed to become an exponential distribution only with \lambda = 1/2 parameter with one step but to be r = 9 for one step.