Answer:
the work done in pulling the bucket to the top of the well is 2125 ft-lb
Explanation:
Given that;
Weight of the bucket is 3 lb
weight of water which can be filled in the bucket is 42 lb
Total weight of bucket and water = 3 + 42 = 45 lb
distance, the bucket filled with water is to be pulled 50 ft
now, let at any time t be the bucket at distance x ft from the bottom of the well
then, t = x/2 × S
where S is the rate at which water is leaking from the bucket
so at this time t, the amount pf water which leaked from the bucket is;
⇒ x ft / 2.5 ft/s × 0.25 lb/s
= 0.25 lb.s⁻¹ / 2.5 ft.s⁻¹ × x ft
= 0.1 lb/ft × x ft
= 0.1x lb
now, as x represent the distance that the bucket has been raised, we the force F applied to the bucket x to be;
F = ( 45 - 0.1x ) lb
so, the required worked by Riemann sum as;
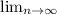 ⁿ∑_
ⁿ∑_
 ( 45 - 0.1x
( 45 - 0.1x
 * ) Δx
* ) Δx
so, the work done, pulling the bucket up will be;
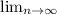 ⁿ∑_
ⁿ∑_
 ( 45 - 0.1x
( 45 - 0.1x
 * ) Δx =
* ) Δx =
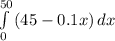
= [ 45x - 0.1
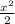 ]⁵⁰₀
]⁵⁰₀
= 45 × 50 - 0.1/2 × (50)²
= 2250 - 125
= 2125 ft-lb
Therefore, the work done in pulling the bucket to the top of the well is 2125 ft-lb