Question

new type of cell phone is being released to the public. The function below is used to predict f(t), the total sales in thousands of units per day based on t, the time in weeks since the date of release. f (t)= 40 (1.18) t According to this function, what are the predicted sales on the first day, and what is the predicted rate of percentage increase per week in the sales amount? 40,000 units on the first day then 1.18% per week 118,000 units on the first day then 40% per week 18,000 units on the first day then 40% per week 40,000 units on the first day then 18% per week

Answer 1

40,000 units on the first day then 18% per week

Explanation:

Exponential function:

An exponential function has the following format:

`f(t) = f(0)(1+r)^(t)`

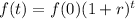
In which r is the rate of change, as a decimal.

In this question:

The number of phones sold in thousands of units per day, in t weeks since the date of release, is:

`f(t) = 40(1.18)^(t)`

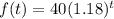
Since
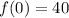`f(0) = 40`, it solds 40,000 on the first week.

The rate of percentage increase is:

1 + r = 1.18

r = 0.18

So an increase of 0.18 = 18% a week.

So the correct option is:

40,000 units on the first day then 18% per week