Question

You work in the packaging department of a company that makes soup. The old soup can is 8 inches high and has a diameter of 4 inches.

Your department is asked to design a bigger soup can that will hold one-and-a-half times as much soup as the old can. Find the height of the bigger soup can if the diameter stays the same.

Answer 1

12 inches high

Explanation:

• Volume of a cylinder =
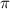  `\pi`r²h
  (where r = radius and h = height)
  
• Radius r = 1/2 diameter

First, find the volume of the original can of soup by using the volume of a cylinder formula and r = 2:

Volume of original can of soup =
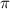`\pi` x 2² x 8 = 32
`\pi`

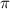
If the new soup can needs to hold 1.5 times as much soup as the original can, multiply the volume of the original can by 1.5:

volume of new can = 32
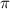`\pi` x 1.5 = 48
`\pi`

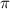
If the diameter of the new can is the same as the diameter of the original can, then r = 2.

Use the volume of a cylinder formula, set the volume to 48
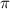`\pi` and use r = 2. Solve for h:

48
`\pi` =
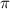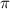`\pi`2²h

Divide both sides by
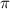`\pi`: 48 = 4h

Divide both sides by 4: 12 = h

Therefore the height of the new can will be 12 in