Question

An investor deposits $20,000 in a bank account that offers an APR 7.9% .How many years will take the investment to double ?

Answer 1

The number of years it will take for the investment to double is approximately 9.1162 years (9 years, 1 month and 12 days)

Step-by-step explanation:

The principal amount the investor deposits in the bank, P = $20,000.00

The annual percentage rate the bank applies to the principal, r = 7.9%

The amount, 'A', in the bank account after a given number of years, 't', is given as follows;

`A = P \cdot \left(1 + (r)/(n) \right)^(n\cdot t)`

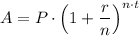
Where;

n = The number of times the interest rate is applied per time period = 1

When the investment (principal amount) doubles, we have;

A = 2·P

r = 0.079

P = $20,000

Plugging in the values into the equation that gives that amount in the account after 't' years, we get;

`2\cdot P = P \cdot \left(1 + (r)/(n) \right)^(n\cdot t)`

`2 = \left(1 + (r)/(n) \right)^(n\cdot t)`

`2 = \left(1 + (0.079)/(1) \right)^(1* t)`

`\therefore 2 = \left(1 .079}{1} \right)^( t)`

`t = (ln(2))/(ln(1.079)) =(196372)/(21541) \approx 9.1162 \, years`

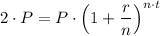
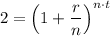
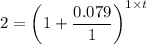
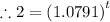
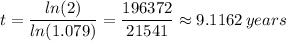
Therefore, it would take t ≈ 9.1162 years for the investment to double