Hello!
For this type of problem, we are given a right triangle, and my go-to for finding a side length with another given side length and an angle value would most likely be the law of sines.
The law of sines states that:
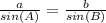
In the given triangle, the
 would be 45, and the opposite angle
would be 45, and the opposite angle
 , would be the
, would be the
 .
.
The same can be applied to the other side of the proportion.

The opposite angle of side
 can be found using the definition of the combined angle of a triangle.
can be found using the definition of the combined angle of a triangle.
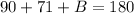
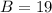
So now we can set up our proportion.
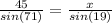
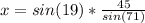
And we get that
 is around 15.5.
is around 15.5.
Hope this helps!