Question

4/4 pts

***
---the system of simoultanious equation
X+2y+z=6
2x+y+2z=6
X+y+z=5​

Answer 1

The system of equations has no solution.

Explanation:

Let the following system of linear equations:

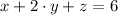`x +2\cdot y + z = 6` (1)

`2\cdot x + y + 2\cdot z = 6` (2)

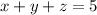`x + y + z = 5` (3)

Since the quantity of equations and variables are the same, there is only one solution to this system. First, we clear
`x` in (3):

`x = 5 - y - z`

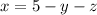
And make substitution both in (1) and (2):

`(5-y-z) +2\cdot y + z = 6`

`2\cdot (5-y-z)+y+2\cdot z = 6`

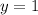`y = 1` (4)

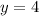`y = 4` (5)

Which lead to a contradiction, since
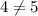`4\\e 5`. Therefore, the system of linear equations has no solution.