Question

Triangle abc is similar to triangle def solve for both unknown sides

Answer 1

Given:

Triangles ABC and DEF are similar triangles.

AB = 6 m, BC = 16 m, CA = 15 m, DE = x, EF = 32 m, FD = y

To find:

The values of unknown sides, i.e., x and y.

Solution:

We know that the corresponding parts of similar triangles are proportional and triangles ABC and DEF are similar triangles, so

`(AB)/(DE)=(BC)/(EF)=(CA)/(FD)`

`(6)/(x)=(16)/(32)=(15)/(y)`

`(6)/(x)=(1)/(2)=(15)/(y)`

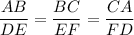
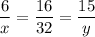
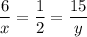
Now,

`(6)/(x)=(1)/(2)`

`6* 2=1* x`

`12=x`

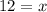
Similarly,

`(1)/(2)=(15)/(y)`

`1* y=15* 2`

`y=30`

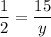
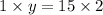
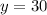
Therefore, the measure of unknown sides are x = 12 m and y = 30 m.