Question

16

10
Use soh-cah-toa to determine the measure of the
indicated angle:
е
B.
.028°
58
39°
51°

Answer 1

58°

Explanation:

We will use the tangent function since we know the opposite and adjacent sides.

Tangent = opposite/adjacent

Tan(e) = 16/10

Tan(e) = 1.6

Use the inverse tangent function to find the angle.

Arctan (1.6) = 57.9946168

Rounding this we get: 58°

Answer 2

`\boxed {\boxed {\sf 58 \textdegree}}`

Explanation:

The three trigonometric ratios (Soh- Cah- Toa) are:

• sin(θ) = opposite/hypotenuse
• cos(θ)= adjacent/hypotenuse
• tan(θ)=opposite/adjacent

We want to find the indicated angle. We have the opposite side (16) and the adjacent (10). Therefore, we must use tangent.

`tan(\theta)= (opposite)/(adjacent)`

`tan(\theta)=(16)/(10)`

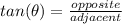
To find an angle measure, the inverse tangent must be used.

`\theta= tan^(-1) ((16)/(10))`

Type the right side into a calculator.

`\theta=57.99461679`

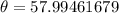
If this is rounded to the nearest whole number, the 9 in the tenth place tells us to round the 7 up to a 8.

`\theta \approx 58`

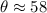
The angle is about 58 degrees.