Answer:
a. Function: Yes
Domain = 1, 5, 10, 11, 14
Range = 6, 50, 150, 176, 266
b. Function: Yes
Domain = All positive integers
Range = All positive integers
c. Function: The relation is not a function
Explanation:
A function is a relation where each input value has only one output value
a. The given data are presented as follows

For each value of the input variable, the width, there is exactly one out put variable, therefore, the relation is a function
Function; Yes
The domain, the input values, includes; 1, 5, 10, 11, 14
The range, the output, values, includes; 6, 50, 150, 176, 266
b. Hours worked versus salary

For each value of the input variable, hours worked, there is exactly one out put variable, therefore, the relation is a function with the following equation;
Salary = $30 × Hours worked
Function; Yes
The domain, the input values, includes; All positive integers
The range, the output, values, includes; All positive integers
c. Height versus age
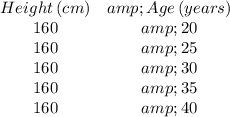
For each value of the input variable, the height, there are more than one out put variable, the age, therefore, the relation is not a function
Function; No