Answer:
4 inches.
Explanation:
Let l = length, w = width, and h = height
The formula for the volume of a rectangular solid would be V = lwh. We are tasked with finding the length of the delivery box. In order to find the length, we need to change the variables so that l is the only variable in the equation.
The prompt tells us that the width of the box is 3 inches longer than the length. That means that w = l + 3. Likewise, the prompt also informs us that the height is 4 inches longer than the length. Therefore, h = l + 4.
Now that the variables have been changed so that l is the only variable, we can finally solve for l.
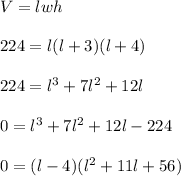
After factoring, we are left with (l - 4)(l² + 11l + 56) = 0. Ultimately, by using the quadratic formula, l² + 11l + 56 = 0 will have imaginary number solutions. However, l - 4 = 0 gives us l = 4. Therefore, the length of the delivery box is 4 inches.