Answer:
It can not be modeled by a linear function
Explanation:
The given parameters can be represented as:

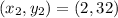
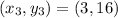
Where: x = rounds and y = players
Required:
Determine if it can be represented by a linear function
To do this, we simply calculate the slope (m)

and
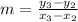
Using:
 , we have:
, we have:
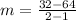
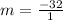
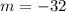
Using
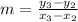 , we have:
, we have:
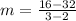

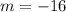
Since both slopes are not the same, the relationship be modeled by a linear function