Question

A G.P is such that the third term is nine times the first term, while the second term is one twenty fourth of the fifth term findits fourth term

Answer 1

Not a G.P

Explanation:

Given - A G.P is such that the third term is nine times the first term, while the second term is one twenty fourth of the fifth term

To find - find its fourth term.

Proof -

Let first term of G.P = a

Second term of G.P = b

Third term of G.P = c

Fourth term of G.P = d

Fifth term of G.P = e

Now,

Given that the third term is nine times the first term

⇒c = 9a

And

Given that the second term is one twenty fourth of the fifth term

⇒b =
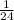`(1)/(24)` e

We know that in a g.P, there is common ratio in the terms

i.e
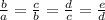`(b)/(a) = (c)/(b) = (d)/(c) = (e)/(d)` = r

and the nth term is represented as aₙ = arⁿ⁻¹

Now,

Second term would be b = ar

Third term would be c = ar²

fourth term would be d = ar³

Fifth term would be e = ar⁴

Now,

c = 9a

⇒ar² = 9a

⇒r² = 9

⇒r = 3 or -3

And

b =
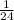`(1)/(24)` e

⇒24b = e

⇒24(ar) = ar⁴

⇒24 = r³

As we have r = 3 or -3

S0 (3)³ = 27 ≠ 24

or (-3)³ = 27 ≠ 24

It is not possible.

So, it is not a G.P