Question

The x-intercept of the function f(x)=x^2-81

Answer 1

hendoebhdjdbdjjsjsnsnns

jskebeuejbeiwnwvgwvshcc

Answer 2

x=9

x=-9

Explanation:

The x intercept or roots are x values that makes the f(x) equal 0. So in order words, to find the x intercept. Set the equation equal 0.

`{x}^(2) - 81 = 0`

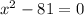
We can approach this problem 2 ways

Way 1: Differnece of Squares

Recall that

`a {}^(2) - {b}^(2) = (a + b)(a - b)`

Since x^2 and 81 are both perfect squares and 81 is negative, we can say that

`(x + 9)(x - 9) = 0`

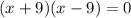
Set each equation to zero

`x = 9`

`x = - 9`

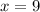
Way 2: Take square root of both sides

`{x}^(2) - 81 = 0`

`{x}^(2) = 81`

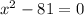
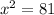
Next step, take square root of both sides. Square root produce negative and positive solutions so

`x = 9`

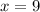
or

`x = - 9`