Question

D)
The size is 22.6 degrees, but how did they get it? ​

Answer 1

Explanation:

a). Since, BC is perpendicular to the plane FEDC, measure of angle BCD = 90°

By applying Pythagoras theorem in ΔBCD,

BD² = BC² + CD²

BD² = (10)² + (24)²

BD = √676

BD = 26 cm

B). Surface area of the wedge = Sum of all the surfaces of the wedge

Area of BCD = Area of AEF =
`(1)/(2)(\text{Base})(\text{Height})`

=
`(1)/(2)(10)(24)`

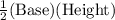
= 120 cm²

Area of CDEF = Length × Width

= ED × CD

= 26 × 24

= 624 cm²

Area of BCFA = 10 × 26 = 260 cm²

Area of ABDE = ED × BD

= 26 × 26

= 676 cm²

Total surface area of the wedge = 2(120) + 624 + 260 + 676

= 1800 cm²

C). Volume of the triangular prism = Area of the triangular base × Height

= (Area of BCD) × DE

= 120 × 26

= 3120 cm³

d). By applying sine rule in the right triangle BCD,

sin(∠BDC) =
`\frac{\text{Opposite side}}{\text{Hypotenuse}}`

=
`(BC)/(BD)`

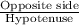
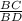
m(∠BDC) =
`\text{sin}^(-1)((10)/(26))`

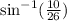
= 22.6°