Question

A motorboat takes 3 hours to travel 108 kilometers going upstream. The return trip takes 2 hours going downstream. What is the rate of the boat in still water and what is the rate of the current?

Rate of the boat in still water:
Rate of the current:

Answer 1

45; 9

Explanation:

1) if the rate of the boat in still water is 'b' and the rate of the current is 'c', then it is possible to write the rate of the boat going upstream: b-c, downstream: b+c.

2) the basic formula is: D=r*t, where D - distance, r - rate/velocity, t - time;

3) according to the condition it takes 3 hours to travel 108 km upstream, it means according to the formula above 3(b-c)=108;

4) according to the condition it takes 2 hours to travel 108 km downstream, it means according to the formula above 2(b+c)=108;

5) if to make up the system of two equations, then:

`\left \{ {{3(b-c)=108} \atop {2(b+c)=108}} \right. \ => \ \left \{ {{b=45} \atop {c=9}} \right.`

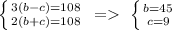
6) rate of the boat in still water: 45 km/h;

rate of the current: 9 km/h.