Question

A bucket that weighs 5 lb and a rope of negligible weight are used to draw water from a well that is 50 ft deep. The bucket is filled with 38 lb of water and is pulled up at a rate of 1.5 ft/s, but water leaks out of a hole in the bucket at a rate of 0.15 lb/s. Find the work done in pulling the bucket to the top of the well. Show how to approximate the required work by a Riemann sum. (Let x be the height in feet above the bottom of the well. Enter xi* as xi.)

Answer 1

Part A

The work done in pulling the bucket to the top of the well is 1,775 ft.·lb

Part B

By Reimann approximation, the work done is 2,130 ft.·lb

Explanation:

Part A

The parameters of the filled bucket of water being pulled upwards are;

The mass of the bucket = 5 lb

The mass of the rope = Negligible

The mass of water that fills the bucket = 38 lb

The rate at which the bucket is pulled up = 1.5 ft./s

The rate at which the bucket leaks, l = 0.15 lb/s

The height in feet above the bottom of the well = x

Therefore, we have;

Height of the bucket, x = 1.5·t

The weight of the bucket after a given time, t, W = 38 - 0.15·t

The time it takes to pull the bucket to the top of the well, t = d/v = 50/1.5 = 100/3 s. =
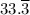`33.\overline3` s

We have;

`W = \int\limits^(t = 33.\overline 3)_(t = 0) {F} \, dx`

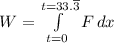
x = 1.5·t, F = Weight of the bucket, W = 38 - 0.15·t

`W = \int\limits^(t = 33.\overline 3)_(t = 0) {(38-0.15\cdot t)} \, \cdot 1.5\cdot dt = \int\limits^(t = 33.\overline 3)_(t = 0) {(57-0.225\cdot t)} \, dt`

`W = [57\cdot t - 0.225 \cdot t^2]_0^(33.\overline 3)`

Therefore, we have;

W = (57 ×
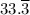`33.\overline3` - (0.225/2) ×
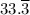`33.\overline3`²) - 0 = 1,775 ft.·lb

The work done in pulling the bucket to the top of the well, W = 1,775 ft.·lb

Part B

By summing the areas of the rectangular areas on the graph, we have

W = 10 × 38 + 10 × (38 - 0.1×10) + 10 × (38 - 0.1 × 20) + 10 × (38 - 0.1 × 30) + 10 × (38 - 0.1 × 40) + 10 × (38 - 0.1 × 50) = 2,130

Therefore, by left Reimann approximation, the work done, W = 2,130 ft.·lb

(More precisely, we have;

The weight at the bottom, F₁ = 38 lb.

The weight at the top of the well, F₂ = 38 - 0.15 × 100/3 = 33

∴ F₂ = 33 lb.

The average weight,
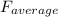`F_(average)` = (F₁ + F₂)/2 = (38 lb. + 33 lb.)/2 = 35.5 lb.

Therefore, the work done,
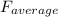`F_(average)` × d = 35.5 lb × 50 ft. = 1,775 ft.lb

The work done, W = 1,775 ft.lb)