Question

Mercury(II) oxide decomposes to form mercury and oxygen, like this:

2Hg (l) + O2 (g) → 2HgO (s)
At a certain temperature, a chemist finds that a reaction vessel containing a mixture of mercury(II) oxide, mercury, and oxygen at equilibrium has the following composition:
compound amount
Hg 14.7g
O2 13.4g
HgO 17.8g
Calculate the value of the equilibrium constant for this reaction. Round your answer to significant digits. Clears your work. Undoes your last action. Provides information about entering answers.

Answer 1

For the reaction given :

`$\text{2Hg}_((l))+\text{O}_2_((g)) \rightarrow \text{2HgO}_((s))$`

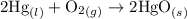
Thus we know that the equilibrium constant
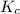`$K_c$` contains aqueous an dgas species only.

∴
`$K_c=(1)/([O_2])$` ............(1)

Now at the equilibrium, an amount of the 13.4 g of oxygen was found in the vessel of 6.9 liters. For determining the concentration of the oxygen gas, we use :

`$[O_2]= (n_(O_2))/(V_(soln))$` ................... (2)

Here,
`$n_(O_2)$` = no. of moles of oxygen gas (mol)

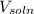`$V_(soln)$` = volume of solution (L)

Therefore the number of moles of the oxygen gas is calculated by directly using the molecular weight (31.9988 g/mol) as the conversion factor.

∴
`$n_(O_2)= 13.4 \ g * \frac{\text{1 mol}}{31.9988 \ g}$`

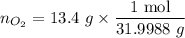
= 0.418 mol

Now substituting the known values in (2), we can find the equilibrium concentration of the oxygen gas :

`$[O_2] =\frac{0.418 \ \text{mol}}{6.9 \ \text{L}}$`

= 0.0605 M

Therefore substituting the result in (1), the equilibrium constant for the reaction is :

`$K_c=(1)/(0.0605)$`

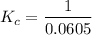
= 16.52