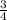Answer:
A.
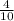
B.
Explanation:
Given - Box A contains 3 red and 2 blue marbles while Box B contains 2
red and 8 blue marbles. A fair coin is tossed. If the coin turns up
heads, a marble is chosen from Box A; if it turns up tails, a marble
is chosen from Box B.
To find - A. Find the probability that a red marble is chosen.
B. Referring to above information, suppose that the person who
tosses the coin does not reveal whether it has turned up
heads or tails (so that the box from which a marble was
chosen is not revealed) but does reveal that a red marble was
chosen. What is the probability that Box A was chosen (i.e.,
the coin turned up heads)?
Proof -
As given ,
Box A : 3 red marbles and 2 blue marbles.
Box B : 2 red marbles and 8 blue marbles.
a.)
As we have to find the probability that a red marble is chosen.
A red marble is chosen in two different ways - either it can be from Box A or Box be .
If Box A is chosen , then Probability of red ball is

If Box B is chosen , Then Probability of Red ball is
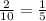
Now,
Probability of Box A is Chosen =

Probability of Box B is chosen =

∴ we get
Probability that a Red marble is chosen =
 ×
×
 +
+
 ×
×
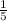 =
=
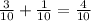 = 0.4
= 0.4
b.)
Now,
Probability that Box A is chosen given that Red marble is chosen is
P(Box A | Red marble ) =
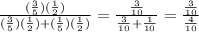 =
=