Answer:
Explanation:
I'm assuming that strange looking thing is a 9, so begin by multiplying both sides by that denominator to get:
 then subtract 3 from both sides to get
then subtract 3 from both sides to get
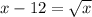 and then square both sides to get
and then square both sides to get
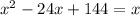 and get everything on one side to solve for x by factoring:
and get everything on one side to solve for x by factoring:
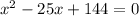 and factor that however it is you have learned to factor second-degree polynomials to get that
and factor that however it is you have learned to factor second-degree polynomials to get that
x = 16 and x = 9. We have to check for extraneous solutions because anytime you manipulate an equation, as we did by squaring both sides, you run the risk of these solutions that actually don't work when you plug them back into the original equation. Let's try 16 first:
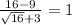 If 16 is a solution, then this statement will be mathematically true.
If 16 is a solution, then this statement will be mathematically true.
 and
and
 so 16 works. Let's try 9:
so 16 works. Let's try 9:
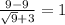 We know that one doesn't work, because 9-9 = 0 and 0 over anything = 0, not 1.
We know that one doesn't work, because 9-9 = 0 and 0 over anything = 0, not 1.
x = 16 is the only solution.