1 Answer

Tonny Xu · Answer 1 · 2022-01-16T23:08:50+0000

Given:

AD is an angle bisector in triangle ABC.
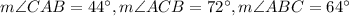$m\angle CAB=44^\circ, m\angle ACB=72^\circ, m\angle ABC=64^\circ$ .

To find:

The value of
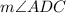$m\angle ADC$ .

Solution:

AD is an angle bisector in triangle ABC.

$m\angle CAD=m\angle BAD=(m\angle CAB)/(2)$

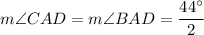
$m\angle CAD=m\angle BAD=(44^\circ)/(2)$

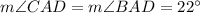
$m\angle CAD=m\angle BAD=22^\circ$

According to the angle sum property, the sum of all interior angles of a triangle is 180 degrees.

Using angle sum property in triangle CAD, we get

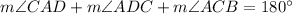
$m\angle CAD+m\angle ADC+m\angle ACB=180^\circ$

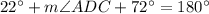
$22^\circ+m\angle ADC+72^\circ=180^\circ$

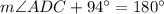
$m\angle ADC+94^\circ=180^\circ$

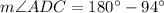
$m\angle ADC=180^\circ-94^\circ$

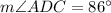
$m\angle ADC=86^\circ$

Therefore, the angle of angle ADC is
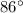$86^\circ$ .

Please log in or register to add a comment.