Answer:
3.12 kg.
Explanation:
Mass after t years:
The mass of the elements after t years is given by the following equation:
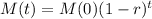
In which M(0) is the initial mass and r is the decay rate, as a decimal.
The half life of Cs-137 is 30.2 years.
This means that:
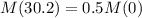
We use this to find r.
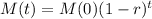
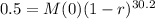
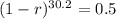
![\sqrt[30.2]{(1-r)^(30.2)} = \sqrt[30.2]{0.5}](https://img.qammunity.org/2022/formulas/mathematics/college/y0dej0ias0xahupx3anw8rm5sw0agmafhk.png)
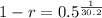
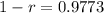
So
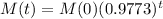
If the initial mass of the sample is 100kg, how much will remain after 151 years?
This is M(151), with M(0) = 100. So
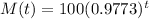
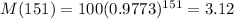
The answer is 3.12 kg.