Given:
The given equation is
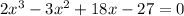
To find:
All the real solutions.
Solution:
We have,
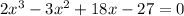
It can be written as
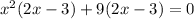
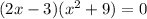
Using zero product property, we get
 and
and
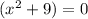
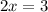 and
and
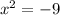
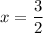 and
and
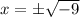
We know that
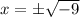 is an imaginary number because there is a negative sign under the square root.
is an imaginary number because there is a negative sign under the square root.
Therefore,
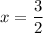 is the only real solution of the given equation.
is the only real solution of the given equation.