Answer:
27 by 27
Explanation:
Let the sides be x and y. The problem is essentially asking:
Given 2(x+y)=108, maximize xy.
We know that x+y=54. By the Arithmetic Mean - Geometric Mean inequality, we can see that
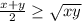 . Substituting in x+y=54, we get
. Substituting in x+y=54, we get
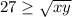 , meaning that
, meaning that
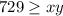 . Equality will only be obtained when x=y (in this case it will generate the maximum for xy), so setting x = y, we can see that x = y = 27. Hence, 27 is the answer you are looking for.
. Equality will only be obtained when x=y (in this case it will generate the maximum for xy), so setting x = y, we can see that x = y = 27. Hence, 27 is the answer you are looking for.